The branch of mathematics which deals with developing and studying methods for analyzing, interpreting, collecting, and presenting data is known as Statistics. This is an important topic for the JEE Main exam. Students are advised to learn important theorems and formulas of statistics so that they can easily solve related problems.
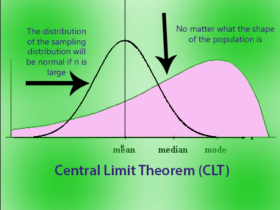
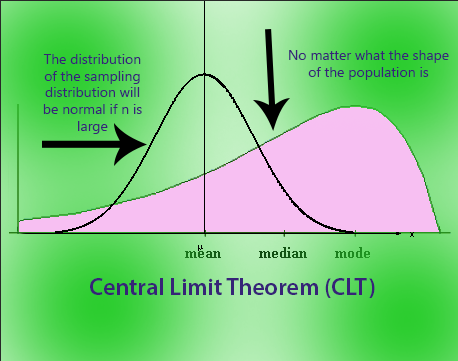
Students can expect 4 marks questions from statistics. Central limit theorem (CLT) is an important statistical theory which states that the samples will be normally distributed and the mean of samples will be approximately equal to the mean of the whole population when the large sample size is having finite variance. This theorem states that for any population with mean μ and standard deviation, the distribution of the sample mean for sample size N has to mean μ and standard deviation σ/√n.
This theory is applied in simplifying analysis with stock index and many more. If the sample size gets bigger, the mean of the sample will come closer to the actual population mean. If the sample is small in size, the actual distribution of the data may or may not be normal. When the sample size gets bigger, it can be approximated by a normal distribution.
Statement
This theorem states that whenever a random sample of size n is taken from any distribution with mean and variance, then the sample means will be approximately normally distributed with mean and variance. The higher the value of the sample size, the better the approximation to the normal.
Assumptions Of CLT
Following are the assumptions of this theorem.
- The sample size should be high .
- The samples should not be dependent on each other.
- The sample should be drawn randomly.
- The sample size should not be more than 10% of the total population, if the sampling is done without replacement.
Formula
The formula is given by
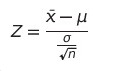
Applications Of CLT
1. The sample mean deviation reduces when we increase the samples taken from the population which helps in estimating the mean of the population more accurately.
2. CLT is used in election polls to find the percentage of people who support a particular candidate as confidence intervals.
3. CLT is used in finding the mean family income.
4. Referring to the sample distribution, CLT can predict whether the sample belongs to a particular population.
Inequalities
Using inequality, we can define the relationship between two unequal values. Inequality means not equal. We use the not equal symbol (≠), to show that two values are not equal. We use different inequalities, to compare the values, whether it is less than or greater than. The symbols used to show inequality are greater than, less than, greater than, or equal to, and less than or equal to. If we relate algebraic expressions using the symbols >, <, ≥, ≤, then we can call the relation an inequality.
While preparing for the JEE exam, students are advised to have a clear understanding of the concept of inequality. This helps them to easily solve related problems and thus score better ranks for the JEE exam.